





COMPASS Preparation
Algebra
Welcome to the online COMPASS preparation module!
My name is Molly Craker and I am an Academic Services Instructor. I have been with CVTC since 2004 and have worked extensivley with students, just like you, to help them prepare for the COMPASS exam. Everything you need to review for the Algebra portion of the COMPASS exam is available on this site. Work your way through the site and visit as often as necessary. If you feel that you would benefit from more help in preparing for the COMPASS, please visit your local CVTC Academic Services. To find the Academic Services closest to you or for additional information please call 715-833-6400.
*The COMPASS test is an entrance exam used by Chippewa Valley Technical College (CVTC) to help place place you in general education courses.
*All students who enter CVTC must take the test.
*The COMPASS test is a computerized test that you schedule to take at your convenience
*COMPASS is an adaptive placement test which means that the test administers questions based on your answers. COMPASS operates at your comfort level rather than your frustration level, by tailoring the items to your skill level, the test will challenge but not discourage. This means that the number of questions and the time to take the test varies for every student.
*You must finish at least 8 questions so that we can determine the classes that you need.
*You are allowed a calculator on both portions of the math test.
*All mathematics items are multiple choice.
*Once you answer a problem you cannot go back to it.
Substituting values into algebraic expressions
Basic operations with polynomials
Solving polynomial equations by factoring
Linear equations in one variable
Linear inequalities in one variable
Setting up equations for given situations
Factoring of polynomials
Formula manipulation
Exponents and radicals
Rational expressions
Systems of linear equations in two variables
Exponents and radicals
Quadratic formulas
Absolute value equations and inequalities
Graphing perpendicular lines
Graphing systems of equations and rational functions
Graphing parallel lines
Midpoint formula
Algebraic expressions are added and subtracted by combining similar terms.
Examples: ( x + y) + (x - y) = x + y + x – y = 2x
(3a2 – 2) - (2a2 – 3a + 4) = 3a2 – 2 – 2a2 + 3a – 4 = a2 + 3a -6
|
|
|
|
|
|
|
|
|
Literal numbers with the same base are multiplied by adding exponents.
(X2) (X3) = X5
A monomial is an algebraic expression having one term. To multiply monomials, we make use of the property of exponents described above:
(2x2)(3x4) = 6x6
|
|
|
|
|
|
|
|
|
Multiply through the parenthesis and then combine like terms, if necessary.
2(x + 3) = 2x + 6
|
|
|
|
|
|
|
|
|
|
|
|
A formula is a brief and clear description of the mathematical relationship between variables. If values can be found for all the literal values in the formula, it can be evaluated.
Example: Area of a trapezoid : ½ h(a + b) h=15, a= 13, b=27
½ (15)(13 + 27) = 300 sq. units
Substitute the given values into the following formulas and solve the resulting expression.
|
|
|
|
|
|
|
|
|
|
|
|
A linear equation is an algebraic equation in which each term is either a constant or the product of a constant and (the first power of) a single variable. If it was graphed, it would form a line.
There is no one fixed procedure which can be applied to solve all linear equations, but the following hints can be offered:
Step 1: Eliminate fractions by multiplying all terms of the equation by the lowest common denominator.
Step 2: Use addition or subtraction to isolate all terms with the unknown on one side of the equation.
Step 3: Combine similar terms on the same side of the equation whenever possible.
Step 4: Divide both sides of the equation by the coefficient of the unknown. Usually this will be the last step in the process.
Examples:
|
3x + 3 = 12 -3 -3 3x = 9 3 3 x = 3
|
2x + 7 = 5x - 5 -2x -2x 7 = 3x - 5 +5 +5 12 = 3x 3 3 4 = x
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
A literal equation differs from other equations because you are not solving for a specific value for a specific variable.
Follow the same steps that were provided for solving linear equations. Solve each equation for the indicated letter.
|
xyz = 3, solve for y
x y z = 3 x z xz
y = 3/xz
|
|
|
|
|
|
|
|
|
|
|
|
|
Line up the two equations vertically so that the x term, the y term and the constant line up. Then add to eliminate one variable. (If one variable doesn't eliminate multiply the entire equation by a number that will make one variable eliminate.) Solve the equation for the remaining variable. Substitute the answer back into one equation and solve for the other variable.
Examples:
|
x + 3y = 11 x - 3y = 5 2x = 16 x = 8
|
2x + 5y = 5 x + 2y = 1 2x + 5y = 5 -2x -4y = -2 (Equation 2 after being multiplied by -2) y = 3
|
|
Substitute into original equation. |
|
|
8 + 3y = 11 -8 -8 3y = 3 y = 1 |
x + 2(3) = 1 x + 6 = 1 x = - 5 |
|
|
|
|
|
|
|
|
|
Exponent Rules:
1. Product Rule
When multiplying terms with the same base, add exponents.
x2 * x3 = x5
2. Quotient Rule
When dividing terms with the same base, subtract exponents.
x5 / x2= x3
3. Power Rule
When you are raising a power to a power, multiply the exponents.
(x3)4 = x12
4. Zero Exponent Rule
Any term or number raised to a power of zero is 1.
( 27x)0 = 1
5. Negative Exponent Rule
Any term that has a negative exponent needs to be rewritten as the reciprocal of the term to eliminate the negative exponent.
x-2 = 1/ x2
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Slope intercept form is written as y = mx + b, where the m indicates the slope of a line and the b indicates the y-intercept or where the line goes through the y-axis. The x-axis is always horizontal and the y-axis is always vertical.
When and equation is not written in the form y = mx + b, it needs to be rewritten in this form using basic algebra rules.
Example:
|
3x + 2y = 10 -3x -3x 2y = -3x + 10 2 2 y = -3/2x + 5
|
y = -3/2 x + 5 So the slope is -3/2 and it goes thought the y-axis at 5.
Write the equation in slope-intercept form.
|
|
|
|
|
|
|
|
Find the slope of each line.
|
|
|
|
|
|
|
|
|
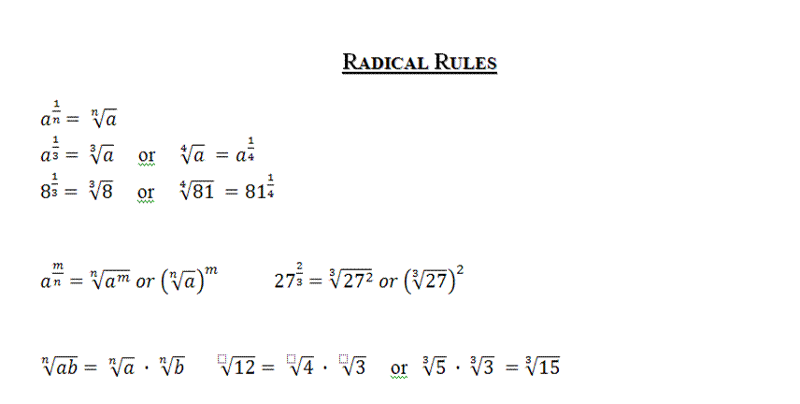


Simplify.
|
|
|
|
|
|
|
|
|
|
|
|
|
|
|
Below are websites where you will find more explanations and additional practice problems. I hope that you have found this module helpful and remember that if you want more help please visit your local CVTC Academic Services.
www.math.com
www.purplemath.com
www.act.org/compass/sample/math.html